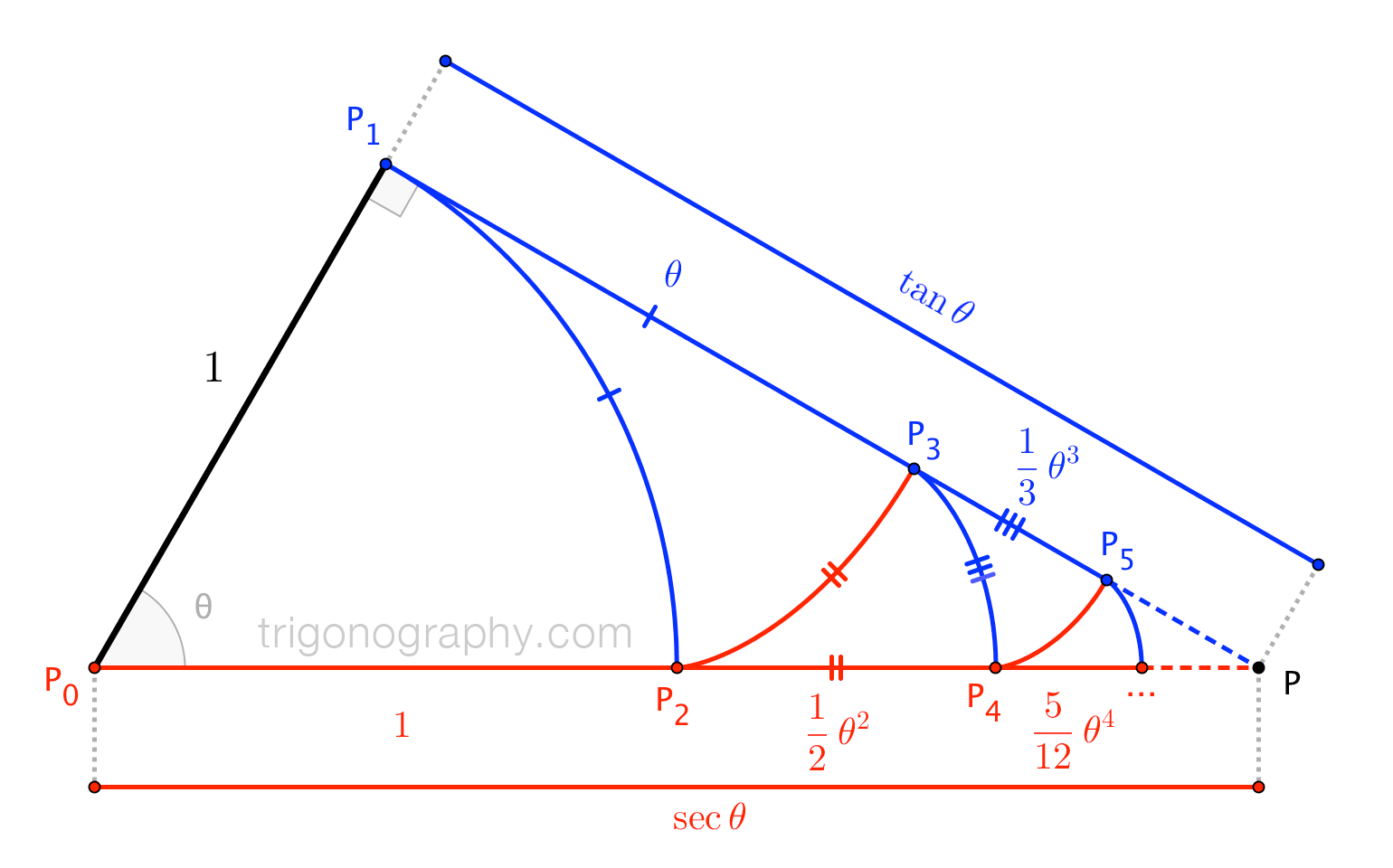
My counterpart to Chaikovsky’s Involute Pinwheel for sine and cosine.
(See “Zig-Zag Involutes, Up-Down Permutations, and Secant and Tangent” (PDF).)
\(\overset{\displaystyle\frown}{P_1P_2}\) is an arc of the unit circle; thereafter, \(\overset{\displaystyle\frown}{P_iP_{i+1}}\) is an involute of \(\overset{\displaystyle\frown}{P_{i-1}P_i}\).
Importantly, the involutes here unfurl in alternating directions,
whereas those in the pinwheel all unfurl the same way.
$$
\begin{align}
\sec\theta &\;=\; |\overline{P_0P_2}| \;+\; |\overline{P_2 P_4}| \;+\; |\overline{P_4P_6}| \;+\; \cdots \\[4pt]
&\;=\; |\overline{P_0P_1}| \;+\; |\overset{\displaystyle\frown}{P_2 P_3}| \;+\; |\overset{\displaystyle\frown}{P_4P_5}| \;+\; \cdots \\[4pt]
&\;=\; \sum_{k=0}^{\infty} \frac{U_{2k}}{(2k)!}\;\theta^{2k} \\[12pt]
\tan\theta &\;=\; |\overline{P_1P_3}| \;+\; |\overline{P_3 P_5}| \;+\; |\overline{P_5P_7}| \;+\; \cdots \\[4pt]
&\;=\; |\overset{\displaystyle\frown}{P_1P_2}| \;+\; |\overset{\displaystyle\frown}{P_3 P_4}| \;+\; |\overset{\displaystyle\frown}{P_5P_6}| \;+\; \cdots \\[4pt]
&\;=\; \sum_{k=0}^{\infty} \frac{U_{2k+1}}{(2k+1)!}\;\theta^{2k+1}
\end{align}$$
where \(U_n\) counts up-down permutations of the set \(\{1, 2, \ldots, n\}\).
\begin{align}
\sec\theta &\;=\; |\overline{P_0P_2}| \;+\; |\overline{P_2 P_4}| \;+\; |\overline{P_4P_6}| \;+\; \cdots \\[4pt]
&\;=\; |\overline{P_0P_1}| \;+\; |\overset{\displaystyle\frown}{P_2 P_3}| \;+\; |\overset{\displaystyle\frown}{P_4P_5}| \;+\; \cdots \\[4pt]
&\;=\; \sum_{k=0}^{\infty} \frac{U_{2k}}{(2k)!}\;\theta^{2k} \\[12pt]
\tan\theta &\;=\; |\overline{P_1P_3}| \;+\; |\overline{P_3 P_5}| \;+\; |\overline{P_5P_7}| \;+\; \cdots \\[4pt]
&\;=\; |\overset{\displaystyle\frown}{P_1P_2}| \;+\; |\overset{\displaystyle\frown}{P_3 P_4}| \;+\; |\overset{\displaystyle\frown}{P_5P_6}| \;+\; \cdots \\[4pt]
&\;=\; \sum_{k=0}^{\infty} \frac{U_{2k+1}}{(2k+1)!}\;\theta^{2k+1}
\end{align}$$
where \(U_n\) counts up-down permutations of the set \(\{1, 2, \ldots, n\}\).
As with the pinwheel, the convergence is more obvious than the numerics.
The referenced note walks through the somewhat-involved combinatorics
of up-down permutations.